Linear Rational Function

Released on 11. 9. 2024 in category SŠ Matematika; Author: Jakub Vojáček; Read count: 125

Introduction to Linear Rational Functions – domain of definition, sketch of the graph, and much more.

A linear rational function is a function of the form `f(x)=\frac{a\mathrm{x}+b}{c\mathrm{x}+d},a, b,c, d \in \mathbb{R}, d \neq 0`

Graph of the function $f(x)=\frac{1}{x}$

The graph of a linear rational function is a hyperbola, and you have already seen its formula. However, there is one detail you need to be aware of. If ad-bc=0 , then the graph of the function is a line with the formula $f(x)=\frac{a}{c}$ .

Properties of the Function

The domain of definition includes all real numbers except one. The denominator must not be zero, so we know that `cx + d \neq 0`. By rearranging this inequality, we find that the domain of the function is: $D_f = \mathbb{R}\backslash\left\{-\frac{d}{c}\right\}$
The range is $H_f = \mathbb{R}\backslash\left\{-\frac{a}{c}\right\}$
The function is unbounded and has neither a maximum nor a minimum.
For , the function is increasing over its entire domain.
For $ad-bc<0$ , the function is decreasing over its entire domain.
The function has two asymptotes: `x=-\frac{d}{c}, y=\frac{a}{c}`

Graph Sketch

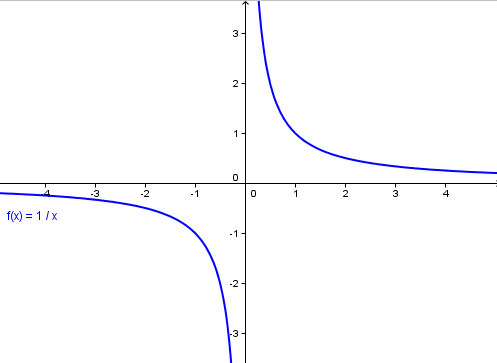
When tasked with sketching the graph of a function, you can calculate its behavior, but this is a long and often difficult process. However, if you recognize that it is a linear rational function, there is a handy method for sketching its graph. If you can transform the function into the form `f(x)=\frac{a}{x+m}+n; a, m, n \in \mathbb{R}`, you can easily sketch its graph.

Converting a linear rational function to this form is straightforward – you simply divide the function into two polynomials:

However, memorizing such a formula is probably unnecessary; it's always easier to simply divide the polynomials.

Convert the function $f(x)=\frac{x+2}{x+4}$ into the other form:

So, we have: $1-\frac{2}{x+4} = \frac{x+2}{x+4}$

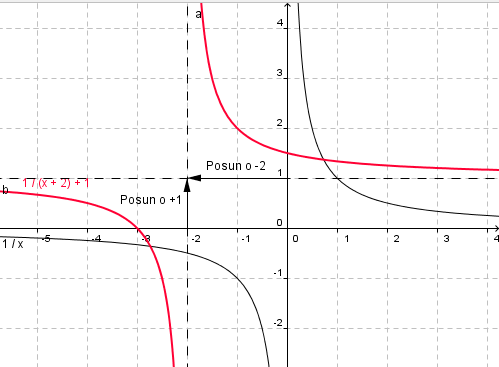
You know how to convert it. Now for the actual graph sketch according to the formula `f(x)=\frac{a}{x+m}+n; a, m, n \in \mathbb{R}`:

If , then the function is increasing. If $a<0$ , the function is decreasing. The parameters determine the shift along the x and y axes. It shifts by along the positive x axis and by along the positive y axis.

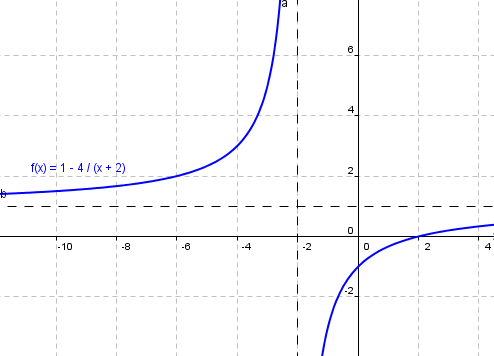
For the function $f(x)=\frac{1}{x+2}+1$ , it looks like this:

Let's try it again. Determine the domain and range of the function $f(x)=\frac{x-2}{x+2}$

The domain and range are obtained simply by substituting into the formula.

`D_f = \mathbb{R}\backslash\left\{-\frac{d}{c}\right\}=\mathbb{R}\backslash\left\{-2\right\}`
$H_f = \mathbb{R}\backslash\left\{-\frac{a}{c}\right\}=\mathbb{R}\backslash\left\{1\right\}$

To sketch the graph, we need to adjust the function:

From this form, you can easily sketch the graph: